LESSON: Human Blood Type and Population Genetics

Today’s lesson is inspired by one of the questions a student asked me earlier this summer.  We’ll be discussing human blood types and population genetics, including how to solve problems on this topic.

The question:

In Capitol City, the allele frequencies of human ABO blood types are as follows:

I^A = 0.2

I^B = 0.3

I^O = 0.5

What is the frequency of type A blood within Capitol City’s population?

There is a lot to know and unpack from this problem.  Let’s start with a quick review of blood types.

Recall that for blood types, A and B refer to the presence of the A and B antigens, respectively, present on red blood cells.  Type A red blood cells express the A antigen; Type B red blood cells express the B antigen.  Type O blood expresses neither A nor B antigen.  That means two genotypes can code for blood that is phenotypically type A:

I^A I^A or I^A I^O

(Why two genotypes?  Because the I^O allele does not code for an antigen.  Instead, the I^O allele contains a mutation that results in a protein that lacks enzymatic activity.)

Similarly, two genotypes can code for blood that is phenotypically type B:

I^B I^B or I^B I^O

Finally, only one genotype can code for blood that is phenotypically type O:

I^O I^O

Now that we’ve established our genotype/phenotype relationships, let’s shift our attention to the second aspect of this question: population genetics.

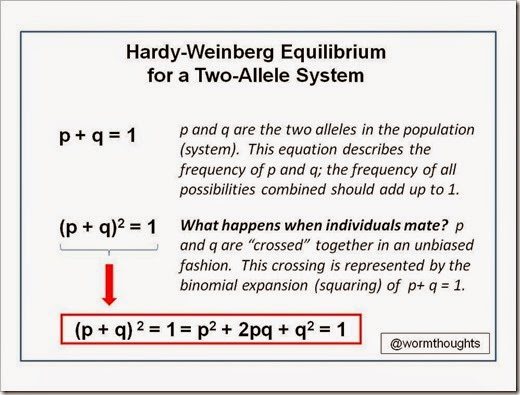
When you see the words “population genetics,” you should immediately think of this phrase: Hardy-Weinberg equilibrium.  The simplest version of Hardy-Weinberg equilibrium is a population that contains two alleles for a gene.  Let’s say these alleles are A and a, where A is completely dominant to a.  In Hardy-Weinberg terms, A and a are equivalent to p and q, the two alleles in our system.  The Hardy-Weinberg equations (shown below) allow us to move between allele frequencies and genotype frequencies.  We use algebra to do these calculations.

To apply Hardy-Weinberg to our two-allele system, A and a, we would have the following:

pp = AA

pq = Aa

qq = aa  

Let’s apply some numbers here to see how the math works out for a two-allele system.  Let’s say that a population has the following frequencies for the A and a alleles:

A = 0.2

a = 0.8

(Note that it’s entirely possible for a recessive allele to be the most common allele in a population.  Genetic dominance does not imply that it’s the most frequently found allele.)

To calculate the frequency of the three possible genotypes (AA, Aa, and aa), we use the binomial expansion from Hardy-Weinberg:

AA = pp = (0.2)(0.2) = 0.04

Aa = 2pq = 2(0.2)(0.8) = 0.32

aa = qq = (0.8)(0.8) = 0.64

Note that our genotype frequencies should add up to 1, which they do!  Success!

Now, let’s turn our attention back to ABO blood types.  We can’t use our two-allele Hardy-Weinberg equation here because we have three alleles.  Instead, we can modify the equations to include a third allele:

By squaring the trinomial, we now have equations we can use to calculate the frequency of particular genotypes or phenotypes if we have the allele frequencies.

Now let’s solve the original problem in four steps.

Alright, now it’s your turn!  Using the data above for Capitol City, what is the frequency of Type AB blood in that population?

* * *

More resources:

* ABO blood types via Wikipedia (a bit dense but still useful for more information)