LESSON: Heat Transfer, Part Two

If you’re new to this site, here is Part One of my lesson on heat transfer.  This lesson will be building on the ideas from Part One.

Now we’ve established that we can calculate values for heat transfer using the formula q = nC[DeltaT].  (Pretend that Delta is a triangle, please.)  Let’s work through a more difficult problem.  This one comes from Chemistry (Third Edition) by John Olsmsted III and Gregory M. Williams.

A silver coin weighing 27.4 g is heated to 100.0 degrees C in boiling water.  It is then dropped into 37.5 g of water initially at 20.5 degrees C.  Find the final temperature of water and coin.

Here is an example of a problem in which we cannot just look at the values given by the problem and perform a plug-and-play calculation.  We are going to use q = nC[DeltaT] but not before we do some algebra work.  Let’s consider the values we have on hand.

Do you see the dilemma?  We have two unknowns, which means we can’t simply plug and play to get an answer.

But notice that we have the same unknowns for the silver coin and water, so perhaps we can use the set of unknowns to set up an algebra equation with a single unknown.

In this problem, we start off with two different systems: the hot silver coin and the room temperature water.  When the coin is dropped into the water, it will transfer heat to the water until the new “system” (coin + water) are at equilibrium, which means SAME TEMPERATURE.  (Also, does “transfer heat” mean anything to you?  If you guessed q, as in heat flow, then you are correct and you get a gold star. )

To put this in mathematical terms:

-q_coin = +q_water   

All the heat that flows out of the coin will be heat that flows into the water.  Furthermore, if q = nC[deltaT], then:

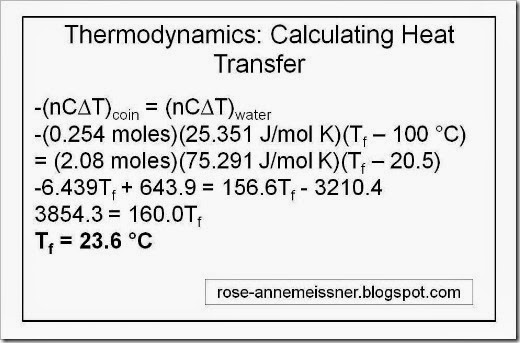
-(nC[deltaT])_coin = (nC[deltaT])_water   

And now we’ve reduced our unknown to one term, final temperature T_f.

Here is the full-length solution, starting from the equation above.

And there you have it!  Now it’s your turn: using the same problem from above, what would the final temperature of water and coin be if the coin were made of pure copper?  The molar heat capacity of copper is 24.435 J/mol K.

(I’ll provide the answer if someone asks for it in the comments.)